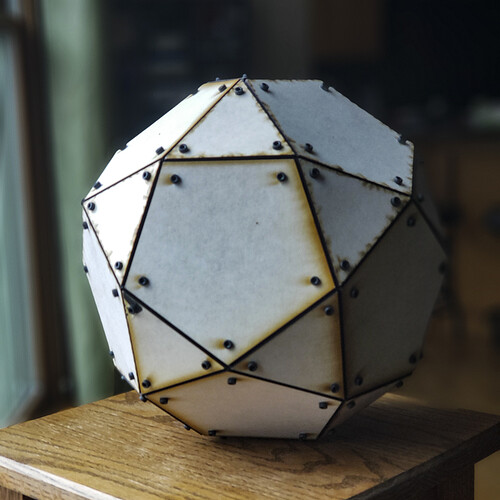
That’s no moon, it’s a Icosidodecahedron.
Here is a bit more information on the build process, so I apologize in advance for such a long post.
I’ve been a big fan of the platonic solids that can be downloaded from Thingiverse, and have created a few Icosadedrons and a couple Dodecahedrons. Making those was one of the reasons I bought a 3D printer, to go alongside my Glowforge. Unlike the Glowforge, where my creations are almost 100% my designs from Illustrator, I hadn’t done much with the 3D stuff, and got most of what I printed off the internet. I forced myself to learn enough Fusion 360 so that I could make the parts necessary to build a Icosidodecahedron. I took a few stabs at it, but finally figured out the math/angles/software to pull it off. I’m sure for 3D guys, it’s simple, but this was new territory for me. The other thing, is that you need to print 30 of these 3D parts to complete the entire shape. It takes my Dremel printer a little over 20 hours on medium to print them in 2 batches of 15.
I used draft board to build a prototype to see if my angles came out correctly. Either by luck, or I might actually know what I’m doing, the thing assembled perfectly. I used 16mm M3 Socket head screws.
View of the inside during construction.
Knowing that my 3D Printed parts would work, I started the 
back up and cut a multi-level design on 
Walnut and Cherry. I went with a Celtic theme.
I’m going o design a stand for this, but will take a break today. I also got Glow in the dark filament from my work Secret Santa, and think that I’ll make one of these in clear Acrylic where the parts glow in the dark on inside!
Want to make one yourself? Buy the Icosidodecahdron Archimedean Solids on Etsy.
Next up: Rhombicosidodecahedron, Rhombicuboctahedron, or Cuboctahedron???
Here’s some info on Icosidodecahedron’s for you geometry nerds:
An icosidodecahedron has icosahedral symmetry, and its first stellation is the compound of a dodecahedron and its dual icosahedron, with the vertices of the icosidodecahedron located at the midpoints of the edges of either.
Its dual polyhedron is the rhombic triacontahedron. An icosidodecahedron can be split along any of six planes to form a pair of pentagonal rotundae, which belong among the Johnson solids.
